Intermediate Algebra
This comprehensive course, combined with the easy-to-use teaching and learning tools in Odigia’s leading learner engagement platform, has everything you need to track, assess, engage, and collaborate with your students. This course comes with content and pre-built assessment questions which can be easily customized or used as-is. Our advanced math editor includes the ability for dynamic and unique variations of questions, as well as the option to customize or create additional practice, quiz, or test questions.

See How Odigia Works

This course includes:

905

dynamic, multi-version questions

Helping Teachers Do What They Do Best: Teach

Customize

Use our courses as is or easily customize them to fit your teaching style and the needs of your students. You can add your favorite resources, hide and show our existing content and pre-built assessments, or make them your own. Everything your students need, in one place!

Engage and Collaborate

Odigia combines learning materials, discussions, and tools to create a familiar social experience for students allowing you to easily connect and redirect students attention.

Track

See how much time students are spending on different areas of the course, which areas are creating the most amount of engagement and identify topics the students are struggling with. Flag and provide feedback on assignments to proactively meet individual students' needs.

Assess

Game theory allows students to monitor their progress visually and motivates them to stay on track. Students can see exactly what activities they need to complete, which ones have been flagged and compare their progress against the overall class.

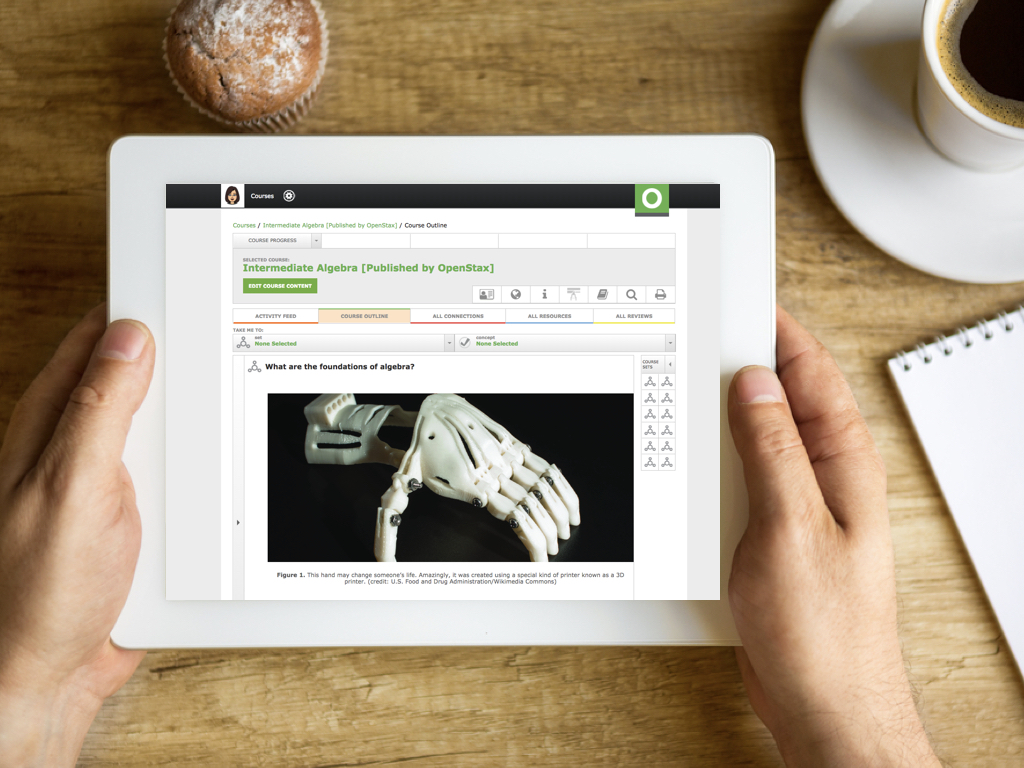
Intermediate Algebra Course Outline

What are the foundations of algebra?

Concepts Covered:

How is the language of algebra used?
What are the basic properties of integers?
How are expressions with fractions simplified and evaluated?
How are problems with decimals solved?
What are the properties of real numbers?

What are the different ways to solve linear equations?

Concepts Covered:

How can a general strategy be used to solve linear equations?
How are problem solving strategies used to solve different types of problems and applications?
How is a formula solved for a specific variable?
How are mixture and uniform motion applications solved?
How are linear inequalities solved?
How are compound inequalities solved?
How are absolute value inequalities solved?

What do the graphs of different types of functions look like?

Concepts Covered:

How are linear equations in two variables graphed?
How is the slope of a line found?
How can the equation of a line be found?
What do the graphs of linear inequalities in two variables look like?
What is the relationship between relations and functions?
What do the graphs of functions look like?

How are systems of linear equations used in real-life situations?

Concepts Covered:

How are systems of linear equations with two variables solved?
How do you solve applications with systems of equations?
How are mixture applications with systems of equations solved?
How are systems of equations with three variables solved?
How are systems of equations solved by using matrices?
How are systems of equations solved by determinants?
What do the graphs of systems of linear inequalities look like?

What are polynomials, and how are they used?

Concepts Covered:

How do you add and subtract polynomials?
What are the properties of exponents and scientific notation?
How do you multiply polynomials?
How do you divide polynomials?

How is factoring applied to real life situations?

Concepts Covered:

How is the greatest common factor of two or more expressions found, and how does factor by grouping work?
What steps must be followed to factor trinomials?
What steps must be followed to factor special products?
What is the general strategy for factoring polynomials?
By what methods can polynomial equations be solved?

How are different operations performed on rational expressions and functions?

Concepts Covered:

What steps must be followed to multiply and divide rational expressions?
How are rational expressions added and subtracted?
How are complex rational expressions simplified?
How are rational equations solved?
How are applications with rational equations solved?
What steps must be followed to solve rational inequalities?

How are expressions with roots and radicals simplified and evaluated?

Concepts Covered:

How are expressions with roots simplified?
How are radical expressions simplified?
How are rational exponents simplified?
How do you add, subtract, and multiply radical expressions?
What steps must be followed to divide radical expressions?
How do you solve radical equations?
How are radicals used in functions?
What is the complex number system, and how is it used?

By what methods are quadratic equations and functions solved?

Concepts Covered:

How are quadratic equations solved by using the square root property?
How are quadratic equations solved by completing the square?
How are quadratic equations solved by using the quadratic formula?
How do you solve quadratic equations in quadratic form?
How are applications of quadratic equations solved?
How are quadratic functions graphed by using properties?
How are quadratic functions graphed by using transformations?
How are quadratic inequalities solved?

What are exponential and logarithmic functions, and how are they solved?

Concepts Covered:

How do you find composite and inverse functions?
How do you evaluate and graph exponential functions?
How do you evaluate and graph logarithmic functions?
How are the properties of logarithms used?
How are exponential and logarithmic equations solved?

What are conics?

Concepts Covered:

What are the distance and midpoint formulas, and how are they used in relation to circles?
How are parabolas graphed and evaluated?
How are ellipses graphed and evaluated?
What are hyperbolas?
How are systems of nonlinear equations solved?

How are sequences, series, and the Binomial Theorem related?

Concepts Covered:

How is the formula of a sequence determined?
How do you determine if a sequence is arithmetic?
How do you determine if a sequence or series is geometric?
What is the Binomial Theorem?

About the book

Intermediate Algebra

Intermediate Algebra is designed to meet the scope and sequence requirements of a one-semester intermediate algebra course. The book’s organization makes it easy to adapt to a variety of course syllabi. The text expands on the fundamental concepts of algebra while addressing the needs of students with diverse backgrounds and learning styles. The material is presented as a sequence of clear steps, building on concepts presented in prealgebra and elementary algebra courses.

This course includes:

905

dynamic, multi-version questions

Helping Teachers Do What They Do Best: Teach

Customize

Engage and Collaborate

Track

Assess

Intermediate Algebra Course Outline

What are the foundations of algebra?

What are the different ways to solve linear equations?

What do the graphs of different types of functions look like?

How are systems of linear equations used in real-life situations?

What are polynomials, and how are they used?

How is factoring applied to real life situations?

How are different operations performed on rational expressions and functions?

How are expressions with roots and radicals simplified and evaluated?

By what methods are quadratic equations and functions solved?

What are exponential and logarithmic functions, and how are they solved?

What are conics?

How are sequences, series, and the Binomial Theorem related?

About the book

Intermediate Algebra

About the authors:

Senior Contributing Authors

Assessment Question Author

Join other subject matter experts who are currently building courses in Odigia.

Share knowledge and expertise while earning extra income.